Hyperbolic
bisector
As
in the Euclidean Geometry we can trace the angle bisector. To use this
tool it will be necessary to give three different
points. The order will be important since with these three points we
will construct an angle with the vertex in the
second given point.
The hyperbolic bisector has to divide an angle into two equal parts. To
construct it we will use the Euclidean bisector. We will also
use the following fact. Given two different Euclidian rays lying on the
same line and with the same origin, if we want
to decide wich ray is nearer from a fixed point we can trace the
perpendicular line for that point. The ray the perpendicular line cuts
will be the nearest. It is because the Euclidean distance from an
exterior point to a line is
found tracing the perpendicular line from the exterior point.
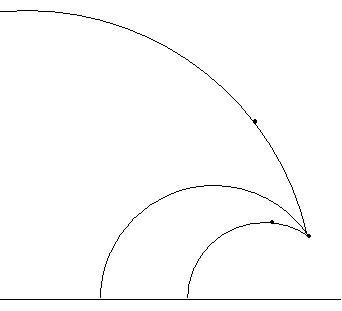
So, to construct the angle bisector we have followed these steps:
- Construct the hyperbolic ray that passes through the two first given points, C and D.
- Construct the hyperbolic ray that passes through the two
last given points, D and E.
- Plot the tangent line, r, to the ray that joins C and D
and that contains D. To plot
the tangent line, we use that the tangent
from a
point in a circumference is the perpendicular line to the
normal one, which is given by the radius. Thus, we plot perpendicular
line to the radius that goes through
point D.
- Draw, as in step (3), the tangent line, s, to the ray that joins D and E
and contains D.
We want to plot the Euclidean bisector of the Euclidean angle,
since we are working in a conformal model. We have to decide which
two of the four Euclidian rays are those we have to consider
since we have had four Euclidean angles. To make it we
use the fact exposed in the first paragraph.
- Plot the perpendicular line to the tangent r that contains C.
- Consider the intersection of the former line with r.
- Plot the Euclidean ray that starts at D and passes through the former
intersection. This ray will
form the Euclidean angle.
- Plot the perpendicular line to s that contains E.
- Consider the intersection of the former
line with s.
- Plot the Euclidean ray that starts at D and passes through the
former intersection. This ray is the other one that
will define the Euclidean angle.
- Plot the Euclidean angle bisector from the point in step
(6),
point D
and the point in step (9).
From the Euclidean angle bisector we will plot the hyperbolic angle
bisector. It should be fulfilled that the Euclidean angle bisector is
tangent to the
hyperbolic one. With this we construct the hyperbolic angle bisector in
the
following way:
- Plot the perpendicular line to the Euclidean
angle bisector that contains D.
Consider the intersection between this line and the boundary
line.
- Plot the circumference with center the intersection in step
(12)
and that passes through D.
The hyperbolic angle bisector we are searching for is on this
hyperbolic line. It only remains to consider three points of the arc
that has to give us
the hyperbolic angle bisector. The initial point is D. The end point is one of the two
intersection points of the circumference with the boundary line.
- Plot the Euclidean segment that joins the
intersection points in steps (6) and (9).
- Consider the intersection point of the segment of the
former step with the Euclidean angle bisector. This intersection exists
because the segment is formed with a point of each ray that defines the
angle we want to bisect.
- Plot the perpendicular line to the Euclidean angle bisector
that passes through
the former point.
- Consider the intersection between the line in step (16) and
the
circumference we have constructed in step (14). The first intersection
point
will be considered as the intermediate point. We consider as the first
point the first found when we go through the line from the
point in step (16).
- To choose the final point, construct the arc of
circumference that passes through D,
the point in step (17) and the antipodal point of D (which we can find tracing
the line that passes through the center of the
circumference and D). The
intersection point of this arc of circumference with the boundary
line is the final point.
- Plot the arc with origin in D, passing through the point in
step (17) and with final point, the point in step (18). This gives us
the
hyperbolic
angle bisector.
To see that the hyperbolic bisector will always be well-constructed it
is necessary to prove that the step (18) will always be well defined,
that is, the perpendicular line will always intersect with the
circumference. We
have already commented during the
construction
the existence of the other intersections. Observe that the steps (7)
and (10) are not necessary for the
construction even though they can be enlighting. In them we construct
the rays that define the Euclidian angle even though this tool only
needs three points (a
point of a ray, the vertex and a point of the other ray). So, with the
intersection
points defined in the steps (6) and (9) we could make the construction.
List of tools
Hyperbolic
geometry